Question

A rectangular garden is 8 feet wide and 15 feet long. A diagonal path cuts through the entire garden. How long is the path?

A)3 feetB)7 feetC)13 feetD)17 feet

Answer 1

`{15}^(2) * {8 }^(2) = 289 \\ \\ √(289 ) = 17`

Answer 2

The path is 17 ft long.

Explanation:

Let c represent the length of the hypotenuse of a right triangle, and let a and b represent the lengths of its legs, as pictured in the image below.

The relationship involving the legs and hypotenuse of the right triangle, given by

`a^2+b^2=c^2`

is called the Pythagorean Theorem.

The diagonal of the rectangle is equivalent to finding the length of the hypotenuse of a right triangle with sides 8 feet wide and 15 feet long.

Applying the Pythagoras' Theorem, we get

`d^2=(8)^2+(15)^2\\d^2=64+225\\d^2=289\\d=√(289)=17`

Technically, there are two answers to
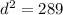`d^2=289`, i.e., d = 17 or d = -17. However, d represents the hypotenuse of the right triangle and must be non-negative. Hence, we must choose d = 17.

The path is 17 ft long.