Question

Factor the polynomial function over the complex numbers.

Answer 1

`[x - 1][x + 1][{x}^(2) + 4]`

Explanation:

Knowing that you have to find two numbers that when they differ to 3, they also multiply to −4, and those numbers are −1 and 4. So, after clearing that up, you will have this [since our degree of this polynomial function is 4]:

`[{x}^(2) - 1][{x}^(2) + 4]`

Then, since
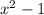`{x}^(2) - 1` is factorable, you will get this:

`[x - 1][x + 1]`

Finally, attach
`[{x}^(2) + 4]` to the partially factored polynomial to get this:

`[x - 1][x + 1][{x}^(2) + 4]`

I am joyous to assist you anytime.