Answer: 14+8.125π units²
Explanation:
By the given diagram,
The diameter of the semicircle = The line segment having the end points (-4,-2) and (3,2),
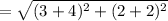
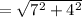
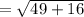
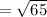 unit,
unit,
Thus, the radius of the semicircle = √65/2 unit,
⇒
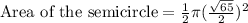
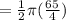
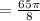 square unit.
square unit.
Now, by the given diagram,
The area of the triangle having the vertices (-4,-2), (3,2) and (-4,2) ( By the coordinate form of area of a triangle formula )
![=(1)/(2)[-4(2-2)+3(2+2)-4(-2-2)]](https://img.qammunity.org/2019/formulas/mathematics/high-school/ml8q0ly7ahi4wmx91p6zeltcxy4yvclmn7.png)
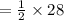
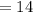 square unit,
square unit,
Hence, the total area of the given figure = Area of semicircle having diameter √65 + area of triangle having vertices (-4,-2), (3,2) and (-4,2)
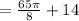
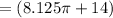 square unit.
square unit.
⇒ Fourth option is correct.