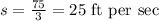Question

The speed of water in a whirlpool varies inversely with the radius. If water speed is 2.5 feet per second at a radius of 30 feet what is the speed of the watte at a radius of 3 feet?

Answer 1

the speed of the water in the 3 feet radius is 25 feet per second.

Answer 2

The speed of the watte at a radius of 3 feet is 25 ft per sec.

Explanation:

Let s represents the speed of water and r represents the radius,

Since, the speed of water in a whirlpool varies inversely with the radius,

`\implies s\propto (1)/(r)`

`\implies s = (k)/(r)------(1)`

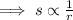
Where, k is the constant of proportionality,

Given,

When, s = 2.5 ft per sec then, r = 30 ft,

So, from equation (1),

`2.5 = (k)/(30)`

`\implies k = 75`

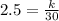
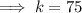
Again from equation (1),

The equation that shows the relation between r and s is,

`s = (75)/(r)`

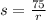
If r = 3 ft,

`s=(75)/(3)=25\text{ ft per sec}`