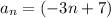Question

HELP PLEASE!!

A recursive rule for an arithmetic sequence is a1=4;an=an−1^−3 .

What is the explicit rule for this sequence?



Enter your answer in the box.

an= ____

Answer 1

The explicit rule for this sequence is -3n+7 I just finished the test.

Answer 2

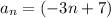`a_(n)=(-3n + 7)` is the answer.

Explanation:

Here the recursive rule for an arithmetic sequence is

a1 = 4

`a_(n)=a_(n-1)-3`

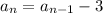
Therefore the sequence will be

4, 1, -2, -5........ n terms

For this arithmetic sequence the explicit rule will be

`a_(n)=a_(1)+(n-1)d`

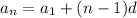
Here n = number of terms

a1 = first term = 4

d = common difference = -3

`a_(n)=4+(n-1)(-3)= 4 - 3(n-1) = 4 - 3n + 3 = (7 - 3n)`

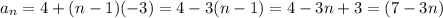
Therefore explicit rule for this sequence is
`a_(n)=(-3n + 7)`