Answer:
The wall area of the room is

Explanation:
I add a graph of the situation in order to explain the question.
In the graph we can see the drawing of the shop room.
I put the measures in the graph.
In order to calculate the wall area of the room, we are going to calculate the area of wall 1, wall 2, wall 3 and wall 4 and then sum between them.
Areas :
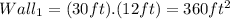

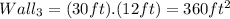
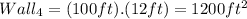
If we sum all the areas :
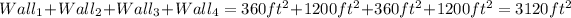
We find that the wall area of the room is
