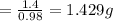Question

In the important industrial process for producing ammonia (the Haber Process), the overall reaction is: N2(g) + 3H2(g) → 2NH3(g) + 24,000 calories A yield of ammonia, NH3, of approximately 98% can be obtained at 200°C and 1,000 atmospheres of pressure. How many grams of N2 must react to form 1.7 grams of ammonia?

Answer 1

28g N2/(17 x 2 x 0.98) g NH3


1.7 g NH3 x 28g N2/(17 x 2 x 0.98) g NH3 =

47.6 / 33.32 = 1.43 g N2

Answer 2

Mass of N2 required = 1.429 g

Step-by-step explanation:

The given reaction is:

N2(g) + 3H2(g) → 2NH3(g)

Mass of NH3 formed = 1.7 g

Molar mass of NH3 = 17 g/mol

`Moles(NH3) = (Mass)/(Molar mass) = (1.7)/(17) = 0. 1`

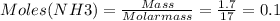
Based on the reaction stoichiometry:

1 mole of N2 forms 2 moles of NH3

Therefore, moles of N2 required to produce 0.1 moles of NH3 is:

`= (1 mole(N2)*0.1moles(NH3))/(2 moles(NH3)) =0.05 moles(N2)`

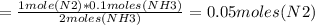
Molar mass of N2 = 28 g/mol

Mass of N2 required = moles*molar mass = 0.05*28 = 1.4 g

This is the theoretical mass corresponding to a 100% yield. Since the yield of NH3 is 98%, the corresponding mass of N2 required would be:

`=(1.4)/(0.98) =1.429 g`