Answer: The correct option is (D) 196608.
Step-by-step explanation: We are given to find the value of the 9th term in the following geometric sequence :
3, 12, 48, 192, . . .
We know that
the n-th term of a geometric sequence with first term a and common ratio r is given by
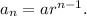
For the given sequence, we have
first term, a = 3 and the common ratio, r is given by
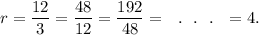
Therefore, the 9th term of the given sequence will be
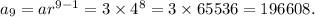
Thus, the required 9th term of the given sequence is 196608.
Option (D) is CORRECT.