Question

The first 3 terms of a sequence are given. Round to the nearest thousandth (if necessary) 460,451,442 find the 49th term

Answer 1

The 49th term of the given sequence

t₄₉ = 28

Explanation:

Step(i):-

Given sequence 460, 451,442,....

first term a = 460

The difference of two terms of given sequence

d = 451 -460 = -9

d = 442 -451 = -9

and so on

The given sequence is in arthematic progression(A.P)

Step(ii):-

The
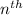`n^(th)` of the given sequence

`t_(n) = a + ( n-1 ) d`

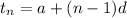
n = 49 , a = 460 and d = -9

The 49th term

`t_(49) = 460 + ( 49-1 ) (-9)`

`t_(49) = 460 + ( 49-1 ) (-9) = 460 -432 = 28`

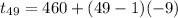
Final answer:-

The 49th term of the given sequence

t₄₉ = 28