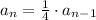Question

What is the recursive rule for this geometric sequence?

−64,−16,−4,−1,...

Enter your answers in the boxes.

Answer 1

`a_n=\left\{\begin{array}{ccc}a_1=-64\\a_(n+1)=a_n:4\end{array}\right\\\\check:\\a_1=-64\\a_2=a_1:4\to a_2=-64:4=-16-OK\\a_3=a_2:4\to a_2=-16:4=-4-OK\\a_4=a_3:4\to a_4=-4:4=-1-OK\\\vdots`

Answer 2

Explanation:

A geometric sequence is defined as the sequence of number that follows a pattern were the next term is found by multiplying by a constant called the common ratio say r.

The recursive rule is given by;

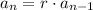`a_n = r \cdot a_(n-1)` where n is the number of terms.

Given the sequence:
`-64, -16, -4 , -1, ....`

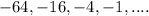
This sequence is a geometric sequence with common ratio (r) =
`(1)/(4)`

Here, first term
`a_1 = -64`

Since,

`(-16)/(-64) = (1)/(4)`

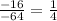
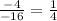`(-4)/(-16) = (1)/(4)` and so on....

The recursive rule for this sequence is;

`a_n = (1)/(4) \cdot a_(n-1)`