For any arbitrary 2x2 matrices
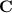
and
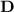
, only one choice of
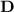
exists to satisfy
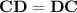
, which is the identity matrix.
There is no other matrix that would work unless we place some more restrictions on
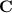
. One such restriction would be to ensure that
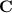
is not singular, or its determinant is non-zero. Then this matrix has an inverse, and taking
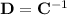
we'd get equality.