Solution :
It is given that about 37% of the adults say cashew nuts are their favorite nut. And a sample of 12 adults are taken to name their favorite nut.
We note that probability of
 successes out of
successes out of
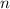 trial is given by :
trial is given by :
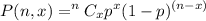
Here, number of trails, n = 12
probability of success, p = 0.37
number of successes, x = 3
a). Therefore the probability of the adults to say cashew nut as their favorite of exactly three is given by :
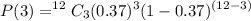
= 0.174217909
b). We know that :
P(at least x) = 1 - P(at most x - 1)
So we use the cumulative binomial distribution table.
i.e.
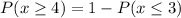
![$= -1[P(x=0)+P(x=1)+P(x=2)+P(x=3)]$](https://img.qammunity.org/2022/formulas/mathematics/high-school/8l59akkzy4f1kgj54nblbre31y8saq624e.png)
![$= 1-[^(12)C_0 (0.37)^0 (0.63)^(12)+^(12)C_1 (0.37)^1(0.63)^(11)+^(12)C_2 (0.37)^2(0.63)^(10)+^(12)C_3(0.37)^3(0.63)^9]$](https://img.qammunity.org/2022/formulas/mathematics/high-school/ilkao7382d2z1ngvt5hvojgg8joimu19bg.png) = 0.70533
= 0.70533
Therefore, P(at least 4) = 0.70533
c).
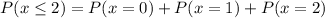
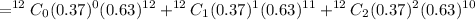
= 0.12045205
Therefore, P(at most 2) = 0.12045205