Answer: There are 220 different possible slates of candidates.
Explanation:
Since we have given that
Total number of members on a board of directors = 12
Number of posts to be elected = 3
As follows:
Chairperson, a secretary, and a treasurer.
So, Number of different slates of candidates that are possible is given by
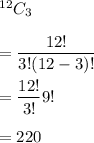
Hence, there are 220 different possible slates of candidates.