Answer:The fact '
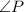 is congruent to itself due to reflexive property' is not used to prove that
is congruent to itself due to reflexive property' is not used to prove that
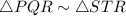
Explanation:
Here we have a triangle PQR in which ST is the line segment which joins the points S and T where
 and
and
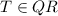
Now let

And, here PR is the common transversal which passes through the parallel lines ST and PQ.
So, In triangles PQR and STR,
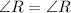 ( reflexive )
( reflexive )
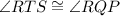 ( by corresponding angle postulate)
( by corresponding angle postulate)
Thus,
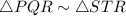 ( by AA postulate of similarity)
( by AA postulate of similarity)
Therefore, from the above proof we can say that except the fact '
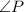 is congruent to itself' we are using all the given options.
is congruent to itself' we are using all the given options.