Question

The equation of a circle whose center is at (1,2) and radius is 5 is

Answer 1

(x-1)^2+(y-2)^2=25
hope this helps!

Answer 2

The answer is
`(x-1)^2+(y-2)^2=25`

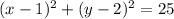
Explanation:

In order to determine the equation of a circle, we have to know the formula about it.

The "standard form equation of a circle" is:

`(x-h)^2+(y-k)^2=r^2`

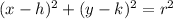
Where:

(h,k)= coordinates of the center of the circle. "h" is the "x" coordinate and "k" is the "y" coordinate.

r=radius of the circle.

So, we just need the center and radius to get the equation of a circle.

In this case:

`(h,k)=(1,2)\\r=5\\\\(x-1)^2+(y-2)^2=(5)^2\\(x-1)^2+(y-2)^2=25`

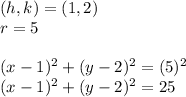
I have attached an image that shows a graph of a circle.