Question

20 POINTS!!!


`(2)/( √(3)cos(x)+sin(x)) =sec (( \pi )/(6) -x)`

Can you help me with this @exordiumx?

Answer 1

`(2)/(\sqrt3\cos x+\sin x)=\sec\left((\pi)/(6)-x\right)\\\\(2)/(\sqrt3\cos x+\sin x)=(1)/(\cos\left((\pi)/(6)-x\right))\ \ \ \ \ (*)\\----------------\\\\\cos\left((\pi)/(6)-x\right)\ \ \ \ |\text{use}\ \cos(x-y)=\cos x\cos y+\sin x\sin y\\\\=\cos(\pi)/(6)\cos x+\sin(\pi)/(6)\sin x=(\sqrt3)/(2)\cos x+(1)/(2)\sin x=(\sqrt3\cos x+\sin x)/(2)\\------------------------------`

`(*)\\R_s=\sec\left((\pi)/(6)-x\right)=(1)/(\cos\left((\pi)/(6)-x\right))=(1)/((\sqrt3\cos x+\sin x)/(2))\\\\=(2)/(\sqrt3\cos x+\sin x)=L_s`

Answer 2

`\ \ (2)/(√(3) \cos (x) + \sin(x)) = \sec\left((\pi)/(6) - x\right)`

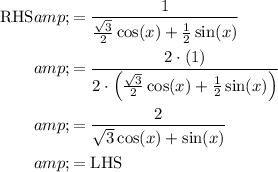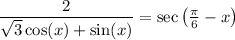
Right-hand side

`\text{RHS} = \sec\left((\pi)/(6) - x\right)`

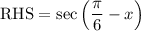
Since
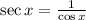`\sec x = (1)/(\cos x)`, it follows that

`\sec\left((\pi)/(6) - x\right) = (1)/(\cos\left((\pi)/(6) - x\right) )`

So we can rewrite

`\begin{aligned} \text{RHS} &= \sec\left((\pi)/(6) - x\right) \\ &= (1)/(\cos\left((\pi)/(6) - x\right)) \end{aligned}`

We have a cosine difference identity for the denominator:

`\begin{aligned} \cos(A-B) &= \cos A \cos B + \sin A \sin B \\ \cos\left(\tfrac{\pi}{6} - x\right) &= \cos\left(\tfrac{\pi}{6}\right) \cos (x) + \sin\left(\tfrac{\pi}{6}\right)\sin(x) \end{aligned}`

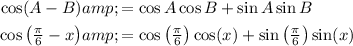
Since
`\sin\left(\tfrac{\pi}{6}\right) = 1/2` and
`\cos\left(\tfrac{\pi}{6}\right) = √(3)/2`, we have

`\begin{aligned}\cos\left(\tfrac{\pi}{6} - x\right) &= \cos\left(\tfrac{\pi}{6}\right) \cos (x) + \sin\left(\tfrac{\pi}{6}\right)\sin(x) \\ &= \tfrac{√(3)}{2}\cos (x) + \tfrac{1}{2}\sin(x) \end{aligned}`

Using this in the right-hand side

`\begin{aligned} \text{RHS} &= (1)/(\cos\left((\pi)/(6) + x\right)) \\ &= \frac{1}{\tfrac{√(3)}{2}\cos (x) + \tfrac{1}{2}\sin(x)} \end{aligned}`

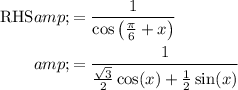
Notice how we have tiny denominators of 2.If we multiply the numerator and denominator of the entire fraction, we will deal with those twos, as 2 will distribute and cancel.

`\begin{aligned} \text{RHS} &= \frac{1}{\tfrac{√(3)}{2}\cos (x) + \tfrac{1}{2}\sin(x)} \\ &=\frac{2 \cdot(1)}{2 \cdot \left(\tfrac{√(3)}{2}\cos (x) + \tfrac{1}{2}\sin(x)\right)} \\ &= (2)/(√(3) \cos (x) + \sin(x)) \\ &= \text{LHS} \end{aligned}`