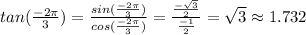Question

What is the value of tan (-2pi/3)?

the correct answer is sqrt 3 but how do i get that? someone explain

Answer 1

`tan((-2\pi)/(3) ) =√(3) \approx1.732`

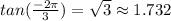
Explanation:

First of all let's define the tangent function:

`tan(\theta )=(Opposite)/(Adjacent) =(sin(\theta))/(cos(\theta))`

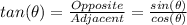
Now, let's define the standard angles, standard angles are those that have values that appear very often in everyday life. These angles are 30°=π/6, 45°=π/4, and 60°=π/3, and the angles 0°, 90°=π/2, 120°= 2π/3, 180°=π, 270°=3π/2, and 360°=2π. The latter, although not defined as 'standard', are also very common. Here are the values:

`cos(0)=1\hspace{25}sin(0)=0\\cos((\pi)/(6) )=(√(3))/(2) \hspace{15}sin((\pi)/(6))=(1)/(2) \\cos((\pi)/(4) )=(√(2))/(2) \hspace{15}sin((\pi)/(4))=(√(2))/(2)`

`cos((\pi)/(3))=(1)/(2)\hspace{28} sin((\pi)/(3) )=(√(3))/(2) \\cos((\pi)/(2))=0\hspace{28} sin((\pi)/(2) )=1}\\cos((2\pi)/(3))=-(1)/(2)\hspace{15} sin((2\pi)/(3) )=(√(3))/(2)`

`cos(\pi)=-1\hspace{25}sin(\pi)=0\\cos((3\pi)/(2) )=0 \hspace{28}sin((3\pi)/(2))=-1 \\cos(2\pi )=1 \hspace{27}sin(2\pi)=0`

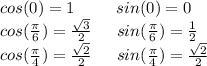
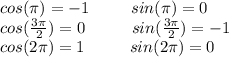
Also you need to keep in mind that cosine function is an even function, and sine function is an odd function, that is:

`cos(-\theta)=cos(\theta)\\\\sin(-\theta)=-sin(\theta)`

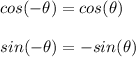
Using these definitions you are able to solve the problem:

`tan((-2\pi)/(3) ) =(sin((-2\pi)/(3) ))/(cos((-2\pi)/(3) )) = ((-√(3) )/(2) )/((-1)/(2) ) = √(3) \approx1.732`

Answer 2

This revolves around exact trig values - no easy way to say this, you just need to memorise them. They are there for sin cos and tan, but I will give you the main tan ones below - note this is RADIANS (always work in them when you can, everything is better):

tan0: 0
tanpi/6: 1/sqrt(3)
tanpi/4: 1
tanpi/3: sqrt(3)
tanpi/2: undefined

Now we just need to equate -2pi/3 to something we understand. 2pi/3 is 1/3 of the way round a circle, so -2pi/3 is 1/3 of the way round the circle going backwards (anticlockwise), so on a diagram we already know it's in the third quadrant of the circle (somewhere between pi and 3pi/2 rads).
We also know it is pi/3 away from pi, so we are looking at sqrt(3) or -sqrt(3) because of those exact values.

Now we just need to work out if it's positive or negative. You can look up a graph of tan and it'll show that the graph intercepts y at (0,0) and has a period of pi rads. Therefore between pi and 3pi/2 rads, the values of tan are positive. Therefore, this gives us our answer of sqrt(3).