Question

A personal trainer designs exercise plans based on a combination of strength-training and aerobic exercise. A beginner plan has 15 minutes per session of strength-training and 20 minutes per session of aerobic exercise for a total of 90 minutes of exercise in a week. An advanced plan has 20 minutes per session of strength-training and 30 minutes of aerobic exercise for a total of 130 minutes of exercise in a week. Which statement describes when the plans are based on the same number of aerobic exercise sessions? Each plan utilizes a combination of 2 strength-training sessions and 2 aerobic exercise sessions per week. Each plan utilizes a combination of 2 strength-training sessions and 3 aerobic exercise sessions per week. Each plan utilizes a combination of 3 strength-training sessions and 2 aerobic exercise sessions per week. Each plan utilizes a combination of 3 strength-training sessions and 3 aerobic exercise sessions per week.

Answer 1

Each plan utilizes a combination of 2 strength-training sessions and 3 aerobic exercise sessions per week.

Explanation:

Let x represent the number of strength-training sessions and y represent the number of aerobic sessions per week.

For the beginner plan, we have

15x+20y = 90. This is because 15 minutes of strength training (x) and 20 minutes of aerobic exercise (y) gives 90 minutes of exercise.

For the advanced plan, we have

20x+30y = 130. This is because 20 minutes of strength training (x) and 30 minutes of aerobic exercise (y) gives 130 minutes of exercise.

This gives us the system

`\left \{ {{15x+20y=90} \atop {20x+30y=130}} \right.`

To solve this, we will make the coefficients of y the same. To do this, we multiply the top equation by 3 and the bottom equation by 2:

`\left \{ {{3(15x+20y=90)} \atop {2(20x+30y=130)}} \right. \\\\\left \{ {{45x+60y=270} \atop {40x+60y=260}} \right.`

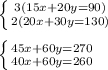
Now to eliminate the y terms, we subtract the second equation from the first:

`\left \{ {{45x+60y=270} \atop {-(40x+60y=260)}} \right. \\\\5x=10`

Divide both sides by 5:

5x/5 = 10/5

x = 2

Substitute this in the first equation:

15(2)+20y=90

30+20y = 90

Subtract 30 from each side:

30+20y-30 = 90-30

20y = 60

Divide both sides by 20:

20y/20 = 60/20

y = 3

Each plan uses 2 strength training sessions (x) and 3 aerobic sessions (y) each week.

Answer 2

From all the statements that are given above the one that best describes when the plans are based on the same number of aerobic exercise sessions is the best answer is each plan utilizes a combination of 2 strength-training sessions and 3 aerobic exercise sessions per week.