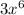Answer:
Option (b) is correct.
The Equivalent expression to given expression
![\sqrt[3]{27x^(18)}](https://img.qammunity.org/2019/formulas/mathematics/middle-school/n424ry1doijai4bbt2dhf5gul9xvfkjzvl.png) is
is
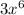
Explanation:
Given : Expression
![\sqrt[3]{27x^(18)}](https://img.qammunity.org/2019/formulas/mathematics/middle-school/n424ry1doijai4bbt2dhf5gul9xvfkjzvl.png)
We have to choose an equivalent expression to the given expression
![\sqrt[3]{27x^(18)}](https://img.qammunity.org/2019/formulas/mathematics/middle-school/n424ry1doijai4bbt2dhf5gul9xvfkjzvl.png)
Consider the given expression
![\sqrt[3]{27x^(18)}](https://img.qammunity.org/2019/formulas/mathematics/middle-school/n424ry1doijai4bbt2dhf5gul9xvfkjzvl.png)
Can be written as

Apply exponent rule
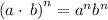
We have,
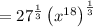
Factor 27 as
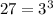
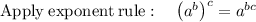
we have,

Also, For
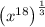
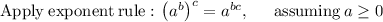
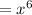
Thus,
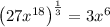
Thus, The Equivalent expression to given expression
![\sqrt[3]{27x^(18)}](https://img.qammunity.org/2019/formulas/mathematics/middle-school/n424ry1doijai4bbt2dhf5gul9xvfkjzvl.png) is
is