Question

Synchronized clocks A and B are at rest in our frame of reference a distance 2 light-minutes apart. Clock C passes A at speed (4/5)c bound for B, when both A and C read t=0 in our frame.

(a) What time does C read when it reaches B?
(b) How far apart are A and B in C's frame?
(c) In C's frame, when A passes C, what time does B read?

Answer 1

a) 89.95 seconds

b) 2.16*10^10 m

c) 149.92 seconds

Step-by-step explanation:

A) The time that C reads when it reaches B

This can be calculated using the equation below

t =
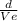`(d)/(Ve)` = (2.16 * 10^10) / (4 * 3 * 10^8)

= 89.95 seconds

where d = 2.16 *10^10 m ( calculated from question b )

b) Determine how far apart A and B are in C's frame

d =
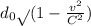`d_(0)\sqrt{} ( 1 - (v^2)/(C^2) )` ---- ( 1 )

do = 3.598 * 10^10 m

Vc = 4/5

hence equation 1 ( d ) = 3/5 * do = 2.16*10^10 m

c) Determine the time that B read when A passes C in C's frame

speed of clock B = 4/5 c

hence time needed by clock B

Tb =
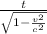`\frac{t}{\sqrt{1-(v^2)/(c^2) } }` ------ ( 2 )

t = 89.95 seconds

v/c = 4/5

input values into equation 2 above

Tb = 149.92 seconds