Question

The pressure exerted by a column of liquid is equal to the product of the height of the column times the gravitational constant times the density of the liquid, p = ghd. how high a column of methanol (d = 0.79 g/ml) would be supported by a pressure that supports a 713 mm column of mercury (d = 13.6 g/ml)?

Answer 1

We know that the pressure at the bottom of the 713 mm column of mercury is equal to an amount of 713 mmHg. The heights will be now, inversely proportional to the densities: So the computation is:(713 mm) (13.63 g/ml) / (0.79 g/ml) = 12,302 mm, or 12.3 meters.

Answer 2

Height, h = 12.27 m

Step-by-step explanation:

The pressure exerted by a column of liquid is equal to the product of the height of the column times the gravitational constant times the density of the liquid i.e.

P = d g h

Pressure, P = 713 mm column of mercury

Density, d = 0.79 g/ml = 790 kg/m³

We have to find how high a column of methanol would be supported by a pressure P. Let the height is h. So,

`h=(P)/(dg)`

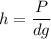
g = acceleration due to gravity 9.8 m/s²

We know that, 1 mm of mercury = 133.32 Pa

713 mm of mercury = 95058.9 Pa

`h=(95058.9\ Pa)/(790\ km/m^3* 9.8\ m/s^2)`

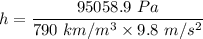
h = 12.27 m

Hence, this is the required solution.