Question

Given: Elmwood St. and Oak Dr. are the same distance. All intersections are perpendicular.

Prove: Peach Tree Dr. is the same distance as Sycamore Ln.

Answer 1

The above result is proved with the help of Pythagoras theorem and explained below.

Explanation:

Given figure ABCD in which AD=BC and all angles intersect at right angle. Hence, the triangle formed inside ADC and ABC are right angled triangle.

In triangle ADC,

By applying Pythagoras theorem, we get

`AC^(2)=AD^(2)+DC^(2)` → (1)

In triangle ABC,

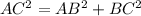`AC^(2)=AB^(2)+BC^(2)` → (2)

Now, from eq (1) and (2)

Peach Tree Dr. is the same distance as Sycamore Ln.

`AD^(2)+DC^(2) = AB^(2)+BC^(2)`

⇒
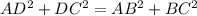
`DC^(2) = AB^(2)` (∵ AD=BC)

⇒ DC=AB (∵Distance can never negative)

Therefore, Peach Tree Dr. is the same distance as Sycamore Ln.