Question

What is the solution of the equation?

Answer 1

`√(2x - 5) = x - 4`

`2x - 5 = (x - 4) {}^(2)`

`2x - 5 = x {}^(2) - 8x + 16`

`x {}^(2) - 10x + 21 = 0`

`(x - 3)(x - 7) = 0`

`x = 3 \\ x = 7`

Answer 2

Answer: 7

Explanation:

`√(2x-5)+4 = x`

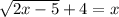
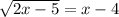`√(2x-5) = x-4` subtracted 4 from both sides

`(√(2x-5))^2 = (x-4)^2` squared both sides to eliminate square root

2x - 5 = x² - 8x + 16 expanded right side

0 = x² - 10x + 21 subtracted 2x and added 5 on both sides

0 = (x - 3) (x - 7) factored right side

0 = x - 3 0 = x - 7 applied zero product property

x = 3 x = 7 solved for x

Check:

x = 3

`√(2(3)-5)+4 = (3)`

`√(1)+4 = 3`

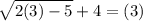
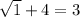
1 + 4 = 3

FALSE! x = 3 is NOT a valid solution

x = 7

`√(2(7)-5)+4 = (7)`

`√(9)+4 = 7`

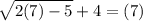
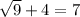
3 + 4 = 7

TRUE! x = 7 IS a valid solution