Question

Aldrin and Jan are standing at the edge of a huge field. At 2:00 p.m., Aldrin begins to walk along a straight path at a speed of 3 km/h. Two hours later, Jan takes a straight path at a 608 angle to Aldrin’s path, walking at 5 km/h. At what time the two friends be 13km apart?

Answer 1

Answer: After 2:29 hours both Aldrin and Jan will be 13 km apart from each other.

Time at that instant will be : 4:00 pm + 2.29 hours= 6:29 pm

Explanation:

According to the figure:

Ab= c = 13 Km

AC = b =
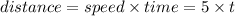`distance= speed* time =5* t`=5t Km

After two hours the distance covered by the Aldrin say till point D :

`distance=speed* time=3km/hr* 2 hrs=6 Km`

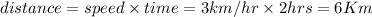
Since, we are interested in determining the time at which Aldrin and Jan both 13 Km apart. For that Aldrin Additional distance covered by the Aldrin from point D to B is :

`distance=speed* time= 3km/hr* t=3t Km`

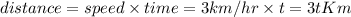
CB= a = CD+DB= 6+3t

We will apply Laws of cosines:

`C^2=A^2+B^2-2AB* Cos\theta`

`(13)^2=(6+3t)^2+(5t)^2-2(5t)(6+3t)Cos 60^o`

`19t^2+6t-133=0`

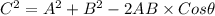
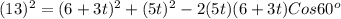
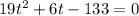
On solving the the above equation by quadratic formula we get two values of 't'

1) t = 2.49 hours , 2) t = -2.80 hours

Since,time cannot be in negative so we will ignore the negative value of t

t = 2.49 = 2:29 hours ,(0.49 hour=0.49 × 60 min=29.4 min)

After 2.49 hours after the walking of Jan. Both Aldrin and Jan will be 13 km apart from each other.

Time at that instant will be : 4:00 pm + 2:29 hours= 6:29 hour