Answer:
Ratio = 3 : 2 and value of m = 5.
Explanation:
We are given the end points ( -3,-1 ) and ( -8,9 ) of a line and a point P = ( -6,m ) divides this line in a particular ratio.
Let us assume that it cuts the line in k : 1 ratio.
Then, the co-ordinates of P =
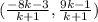 .
.
But,
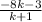 = -6
= -6
i.e. -8k-3 = -6k-6
i.e. -2k = -3
i.e.
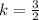
So, the ratio is k : 1 i.e
 i.e. 3 : 2.
i.e. 3 : 2.
Hence, the ratio in which P divides the line is 3 : 2.
Also,
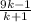 = m where
= m where
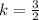
i.e. m =
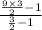
i.e. m =

i.e. m =
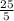
i.e. m = 5.
Hence, the value of m is 5.