Question

Pls help with correct choice

Answer 1

The correct option is 3.

Explanation:

The coordinates of point P are (4,3).

We have to find the value of

`R_(p,90)\circ R_(o,-90):(-1,-1)`

The operations area operated from right to left it means first we have to apply

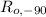`R_(o,-90)`, then
`R_(p,90)`.

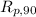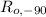`R_(o,-90)` means rotation 90 degree clockwise about the origin, it is defined as

`(x,y)\rightarrrow (y,-x)`

`(-1,-1)=(-1,1)`

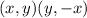
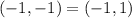
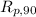`R_(p,90)` means rotation 90 degree counter clockwise about the the point P, it is defined as

`(x,y)\rightarrrow (-(y-3)+4,(x-4)+3)`

`(x,y)\rightarrrow (-y+7,x-1)`

`(-1,1)\rightarrrow (-1+7,-1-1)`

`(-1,1)\rightarrrow (6,-2)`

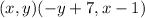
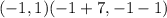
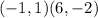
Therefore the coordinates of image are (6,-2). Option 3 is correct.

Answer 2

(-1, -1)

Explanation:

Rotating the point (-1, -1) about the origin at -90° will be a 90 degree clockwise rotation. This will map switch the x- and y-coordinates and negate the x-coordinate:

(x, y)→(y, -x)

(-1, -1) → (-1, 1)

Following this with a 90 degree counter-clockwise rotation will map

(x, y)→(-y, x)

(-1, 1)→(-1, -1)

This is the same point we started with. Thinking about this logically, if we rotate something 90 degrees clockwise and then follow that with a 90 degree counter-clockwise rotation will put the object back in its original position.