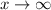Answer: The below graph shows the given function.
Explanation:
Here the given function,

Which is the rational function.
For vertical asymptote,
Denominator = 0
⇒

⇒

⇒
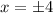
Thus, the vertical asymptotes of the given function are (4, 0) and (-4,0).
Also, x-intercept of the given function are ( 1,0) and (-6,0)
y-intercept of the given function are (0,0.375)
End behavior :
Since, The function has three intervals,
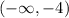

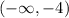
In interval
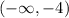 ,
,
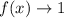 as
as
 and
and
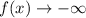 as
as

In interval
 ,
,
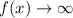 as
as
 and
and
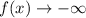 as
as
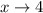
And, In interval
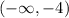 ,
,
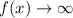 as
as
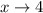 and
and
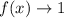 as
as