Question

How are the absolut value of an imaginery number is related distance betweem two point related to another

Answer 1

the absolute value of a imaginary number is somewhat similar to a distance between the two points.

Explanation:

by absolute value of an imaginary number a+i b we mean the distance of the origin (0, 0) and the point (a,b) in the complex plane

i.e.
`\sqrt{a^(2) +b^(2) }`

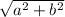
whereas distance between two points (
`x_(1)`,
`x_(2)`) and (
`y_(1)`,
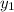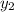`y_(2)`) is given by
`\sqrt{(x_(1)-y_(1) )^2+( x_(2)-y_(2))^2}`

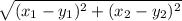
if (
`y_(1)`,
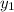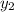`y_(2)`)=(0,0) then it is similar to the absolute value of a complex number of the type x+i y.