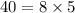Question

An empty kitchen cabinet has 8 shelves and each shelf can hold no more than 30 cans you want to arrange 40 cans in the kitchen cabinet so that the same number of cans is on each shelf that you use

(a) list the factors of 40


(b) list the factors of 40 that represent the number of cans that could be on each shelf


(c) how many different arrangements of cans are possible


(d) what are the possible arrangements of the cans

Answer 1

(a) 40 = 1 x 40, 2 x 20, 4 x 10, or 5 x 8. Factors of 40: 1, 2, 4, 5, 8, 10, 20, 40

(b) Factors: 5, 8, 10, 20

(c) 4, that is, the quantity of factors in (b)

(d) 5 cans in 8 shelves, 8 cans in 5 shelves, 10 cans in 4 shelves, 20 cans in 2 shelves.

Answer 2

(a)

`40=1,2,4,5,8,10,20,40`

(b)

factor=5 can only hold it

(c)

Only one arrangement is possible

(d)

we can put 5 cans into all 8 shelves

(e)

`40=8* 5`

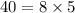
Explanation:

We are given

an empty kitchen cabinet has 8 shelves

and each shelf can hold no more than 30 cans you want to arrange 40 cans in the kitchen cabinet

so that the same number of cans is on each shelf that you use

(a)

we can find all possible factors of 40

`40=1,2,4,5,8,10,20,40`

(b)

Since, an empty kitchen cabinet has 8 shelves

so, one of factor of 40 must be 8

so,

`40=8* 5`

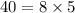
So, factor=5 can only hold it

(c)

Only one arrangement is possible

because there is only one such possible factor

`40=8* 5`

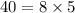
(d)

Since, we got

`40=8* 5`

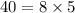
So, we can put 5 cans into all 8 shelves

(e)

Since, there are 8 shelves

and we can put 5 cans on each shelves

so, possible arrangement is

`40=8* 5`