Question

Given the position function s(t)=(t^3/3)-(12t^2/2)+36t between t=0 and t=15, where s is given in feet and t is measured in seconds, find the interval in seconds where the particle is moving to the right.

Answer 1

Answer: [0, 6) ∪ (6, 15]

Explanation:

The particle is moving to the right when the velocity is positive.

`s(t)=(t^3)/(3) -(12t^2)/(2)+36t`

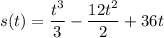
v(t) = s'(t)

= t² - 12t + 36

First, find the zeros of v(t): ⇒ v(t) = 0

0 = t² - 12t + 36

= (t - 6)²

0 = t - 6

6 = t

The zero occurs when t = 6

Next, find when v(t) > 0

Choose a test point between 0 and 6:

v(5) = (5 - 6)²

= (-²)

= (+)

Since velocity is positive between 0 and 6, the particle is moving to the right during that interval.

Choose a test point between 6 and 15:

v(7) = (7 - 6)²

= (+)²

= (+)

Since velocity is positive when t > 6, the particle is moving to the right.