Question

There are 0.3 moles of xenon gas in a 0.5-liter container at 30 degrees C. What is the pressure exerted by the xenon gas

Answer 1

PV = nRT
P = (nRT)/V
P = (0.3 mol × 0.08206 atm-l/(mol-K) × (273.15 + 30) K)/(0.5 l)
P = 14.9258934 atm

Answer 2

Answer : The pressure of the gas is, 14.925 atm

Solution :

using ideal gas equation,

`PV=nRT`

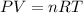
where,

n = number of moles of gas = 0.3 mole

P = pressure of the gas = ?

T = temperature of the gas =
`30^oC=273+30=303K`

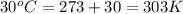
R = gas constant = 0.0821 Latm/moleK

V = volume of the gas = 0.5 L

Now put all the given values in the above equation, we get the pressure of the gas.

`P* (0.5L)=(0.3mole)* (0.0821L.atm/mole.K)* (303K)`

`P=14.925atm`

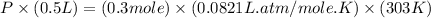
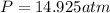
Therefore, the pressure of the gas is, 14.925 atm