Question

If,

a + b = 8,
ab + c + d = 23,
ac + bd = 28, and
cd = 12,
find the values of a, b, c, and d.

Answer 1

Answer: There are four possible solutions

1. a = 3, b = 5, c = 6, d = 2
2. a = 4, b = 4, c = 3, d = 4
3. a = 4, b = 4, c = 4, d = 3
4. a = 5, b = 3, c = 2, d = 6

Explanation:

I was unable to manipulate this system to develop solvable equations, so I made a table consisting of 7 rows with the following 5 columns:

1. a (values of 1 thru 7)
2. b (values of 7 thru 1)
3. a·b (multiplying column 1 by column 2)
4. c + d (subtracting column 3 from 23)
5. cd=12 (two addends from column 4 that create a product of 12)

Table 1

`\begin{array}c{a&b&ab&c+d&cd\\1&7&7&16&\text{null}\\2&6&12&11&\text{null}\\3&5&15&8&2,6\\4&4&16&7&3,4\\5&3&15&8&6,2\\6&2&12&11&\text{null}\\7&1&7&16&\text{null}\\\end{array}`

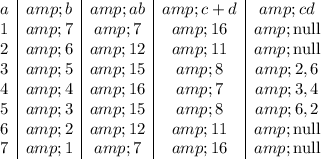
I used the valid solutions (rows 3 thru 5) to determine which combinations satisfied ab + cd = 28.

Table 2

`\begin{array}ca&b&c&d&ab+cd\\3&5&2&6&6+11=17\\3&5&6&2&18+10=28^*\\4&4&3&4&12+16=28^*\\4&4&4&3&16+12=28^*\\5&3&2&6&10+18=28^*\\5&3&6&2&30+6=36\\\end{array}`

* represent the valid solutions