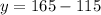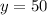Answer:
First man rate is 115
Second man rate is 50
Explanation:
Let's assume rate of charge of first men is x
rate of charge of second men is y
we are given
the sum of the two rates was 165. per hour
so, we get

Two men worked on a car. the first man worked 20 hours and the second man worked 15 hours. Together they charged 3050
so, we get
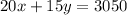
we can simplify it
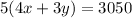
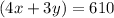
so, we got system of equations as


We can solve for y from first equation and plug into second equation
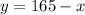
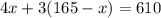
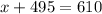
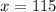
now, we can solve for y