Answer:
Correct choice is C
Explanation:
Triangle Inequality Theorem states that the sum of the lengths of any two sides of a triangle is greater than the length of the third side. Thus, if a, b and c are triangles sides, then
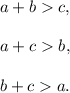
A. Consider lengths a=5, b=10 and c=4. Since
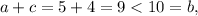
these side lengths couldn't create triangle.
B. Consider lengths a=5, b=7 and c=13. Since

these side lengths couldn't create triangle.
C. Consider lengths a=7, b=9 and c=3. Since
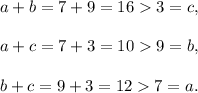
These side lengths could form a triangle.
D. Consider lengths a=10, b=4 and c=5. Since
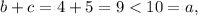
these side lengths couldn't create triangle.