Question

023 (part 1 of 2) 10.0 points

A merry-go-round rotates at the rate of

0.21 rev/s with an 99 kg man standing at

a point 2.8 m from the axis of rotation.

What is the new angular speed when the

man walks to a point 0 m from the center?

Consider the merry-go-round is a solid 53 kg

cylinder of radius of 2.8 m.

Answer in units of rad/s.

024 (part 2 of 2) 10.0 points

What is the change in kinetic energy due to

this movement?

Answer in units of J.

Answer 1

Part 1

The angular speed is approximately 1.31947 rad/s

Part 2

The change in kinetic energy due to the movement is approximately 675.65 J

Step-by-step explanation:

The given parameters are;

The rotation rate of the merry-go-round, n = 0.21 rev/s

The mass of the man on the merry-go-round = 99 kg

The distance of the point the man stands from the axis of rotation = 2.8 m

Part 1

The angular speed, ω = 2·π·n = 2·π × 0.21 rev/s ≈ 1.31947 rad/s

The angular speed is constant through out the axis of rotation

Therefore, when the man walks to a point 0 m from the center, the angular speed ≈ 1.31947 rad/s

Part 2

Given that the kinetic energy of the merry-go-round is constant, the change in kinetic energy, for a change from a radius of of the man from 2.8 m to 0 m, is given as follows;

`\Delta KE_(rotational) = (1)/(2) \cdot I \cdot \omega ^2 = (1)/(2) \cdot m \cdot v ^2`

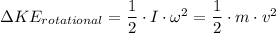
I = m·r²

Where;

m = The mass of the man alone = 99 kg

r = The distance of the point the man stands from the axis, r = 2.8 m

v = The tangential velocity = ω/r

ω ≈ 1.31947 rad/s

Therefore, we have;

I = 99 × 2.8² = 776.16 kg·m²

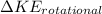`\Delta KE_(rotational)` = 1/2 × 776.16 kg·m² × (1.31947)² ≈ 675.65 J