QUESTION 1
The given polynomial function is

The degree of the polynomial is the exponent on the leading term of the polynomial after the polynomial has been written in descending powers of
 .
.
The leading term is
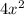 the exponent of
the exponent of
 in this term is
in this term is
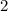 , hence the degree is 2.
, hence the degree is 2.
The coefficient of this term is the leading coefficient which is
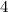 .
.
The correct answer is B.
QUESTION 2
The function graphed has two x intercepts.
The first x intercept has a multiplicity that is even. The least positive even integer is 2.
The second x intercept has a multiplicity that is odd.
The least positive odd integer is 1.
Therefore the lowest degree is the sum of the two least values which is
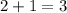
The correct answer is C
QUESTION 3
The given polynomial function is
 , where
, where
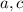 and
and
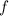 are real numbers.
are real numbers.
The x intercepts are the roots of the polynomial and we know the maximum number of real roots a polynomial of degree 5 can have is 5.
The maximum number of the x-intercepts of the polynomial is therefore 5.
The correct answer is D
QUESTION 4
To find the y-intercept, we substitute
 into each polynomial function.
into each polynomial function.
The first function is

When
 ,
,
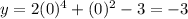
The y-intercept is -3
The second function is
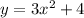 .
.
When
 ,
,
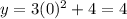 .
.
The y-intercept is 4
The third function is
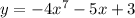 , when
, when
 ,
,
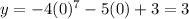
The y-intercept is 3
The fourth function is
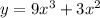
When
 ,
,
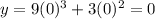
The y-intercept is 0
The correct answer is C.
QUESTION 5
The given polynomial function is
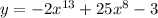 .
.
This is already in standard form.
The degree of the polynomial is 13, which is odd.
The graph of the polynomial will rise at one end and fall at the other end.
The leading coefficient is negative, so the graph rises on the left and falls on the right.
Therefore as x approaches infinity, y will be approaching negative infinity.
The correct answer is D