Answer:
Option C -M'K' is the same length as MK
Explanation:
Given : Line segment MK has endpoints at (2, 3) and (5,4)
M'K' is the reflection of MK over the y-axis
By definition of reflection: reflection of point (x,y) across the the y-axis is the point (-x,y)
which implies M'K' has end points (-2,3) and (-5,4)
Now, we find the length of MK
let
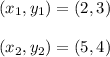
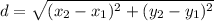
⇒
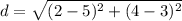
⇒
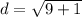
⇒
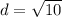 ....(1)
....(1)
Now, we find the length of M'K'
let
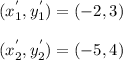
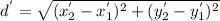
⇒
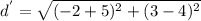
⇒
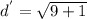
⇒
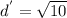 .....(2)
.....(2)
from (1) and (2) we simply show that the length of MK and M'K' is equal
we can also refer the figure attached for reflection of MK and M'K'
therefore, Option C is correct