Answer:
Area = 587877.54

Explanation:
Let the length of sides of the triangle be represented by a, b and c respectively.
a =
 x 3600
x 3600
= 1000
b =
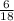 x 3600
x 3600
= 1200
c =
 x 3600
x 3600
= 1400
The length of the sides of the triangle are: 1000 m , 1200 m, 1400 m.
Perimeter = a + b + c
= 1000 + 1200 + 1400
= 3600
The area of the land can be determined by;
Area of a triangle =
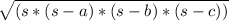
where: s is the semi-perimeter of the triangle and a, b and c are the length of sides respectively.
s =
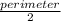
=
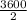
s = 1800
Area =
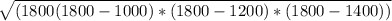
=
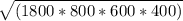
=
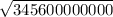
= 587877.54
Area = 587877.54

The area of the triangle is 587877.54
 .
.