Answer: The revenue can be $405 or it can be larger than this value.
In short, the revenue must be at least $405.
======================================
Step-by-step explanation:
Let R be the possible revenue. We want the profit to be at least $250, so we want the profit to be 250 or more
Recall that profit is equal to revenue minus expenses, so
Profit = Revenue - Expenses
Profit = R - 155
The expression R - 155 represents the profit (R is just a placeholder for a number). We want R - 155 to be 250 or larger, so,
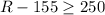
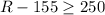
 add 155 to both sides
add 155 to both sides
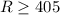
As long as the revenue is $405 or higher, then the profit will be at least $250