Question

On the planet Jupiter, a ball this thrown upward at a velocity at 10m/s. It's height, h(t) meters after t seconds is given by the equation h(t)=-12.5(t-0.4)^2+4.

a) what is the maximum height the ball reaches?


b) what was the height of the ball when it was released from the throwers hand?


c) how many seconds after the ball was hit and did it land on the surface of Jupiter to the nearest second?

Answer 1

a) The maximum height that the ball reaches is 4 meters.

b) The height of the ball when it is released from thrower's hand is 2 meters.

c) The ball will take approximately 0.966 seconds to hit the surface of Jupiter.

Explanation:

a) Let
`h(t) = -12.5\cdot (t-0.4)^(2)+4`, where
`t` and
`h(t)` are the time and height, measured in seconds and meters. Since this equation is in vertex form, the maximum height corresponds to the value associated with the dependent variable (height). That is:

`h(t) -4 = -12.5\cdot (t-0.4)^(2)`

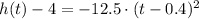
Hence, the maximum height that the ball reaches is 4 meters.

b) The height of the ball when the ball is released from thrower's hand is the height of the ball at
`t = 0\,s`. Then, we evaluate the function:

`h(0) = -12.5\cdot (0-0.4)^(2)+4`

`h(0) = 2\,m`

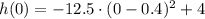
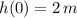
The height of the ball when it is released from thrower's hand is 2 meters.

c) The instants when the ball hits the ground are those instants
`t` so that
`h(t) = 0`. Then:

`-12.5\cdot (t-0.4)^(2)+4 = 0`

`-12.5\cdot (t^(2)-0.8\cdot t +0.16)+4 = 0`

`-12.5\cdot t^(2)+10\cdot t +2 = 0`

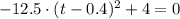
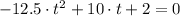
By the Quadratic Formula we obtain the following roots:

`t_(1) \approx 0.966\,s`,
`t_(2)\approx -0.166\,s`

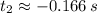
Only the first root is physically reasonable, since time is a positive real variable.

The ball will take approximately 0.966 seconds to hit the surface of Jupiter.