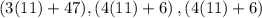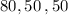Question

Suppose that QRS is isosceles with base SQ . Suppose also that =m∠R+3x47° and =m∠S+4x6° . Find the degree measure of each angle in the triangle.

Answer 1

ANSWER


`m \: < \: R = 80 \degree`


`m \: < \: S=50 \degree`
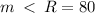
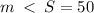
and


`m \: < \: Q = 50 \degree`



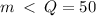
Step-by-step explanation

Since the triangle is an isosceles triangle, the base angles are equal.


The three interiors angles of the triangle are,


`(3x + 47) \degree,(4x + 6) \degree \: ,(4x + 6) \degree`

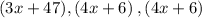
The sum of these three angles must equal 180°.


`(3x + 47) \degree + (4x + 6) \degree \: + (4x + 6) \degree = 180 \degree`


We group like terms to get,


`3x + 4x + 4x = 180 - 47 - 6 - 6`

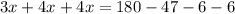
This simplifies to,


`11x = 121`

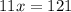
We divide both sides by 11 to get,



`x = 11`


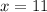
The measure of the angles are,


`(3(11)+ 47) \degree,(4(11) + 6) \degree \: ,(4(11) + 6) \degree`



`(33+ 47) \degree,(44+ 6) \degree \: ,(44 + 6) \degree`



`80\degree,50\degree \:, 50\degree`