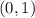Question

What is the y-intercept of f(x)=-X^3+3x^2+1?

Answer 1

ANSWER

The y-intercept is


`(0,1)`


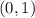
Step-by-step explanation

The given polynomial function is



`f(x) = - {x}^(3) + 3 {x}^(2) + 1`

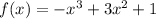
At y-intercept the value of x is zero.


Therefore we need to put

`x = 0`

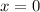
into the given polynomial function to determine the corresponding y-value.




`f(0) = - {(0)}^(3) + 3 {(0)}^(2) + 1`

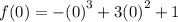
This will give us,



`f(0) = 0 + 0+ 1`

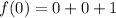
We further simplify to obtain,

`f(0) =1`



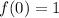
This means that when

`x = 0`
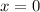
the value of the function is

`1`

Hence the y-intercept is


`(0,1)`