Answer:
407.22 foot is the boat from the base of the lighthouse
Explanation:
Given the statement: An observer on top of a 50-foot tall lighthouse sees a boat at a 7° angle of depression.
Let x foot be the distance of the object(boat) from the base of the lighthouse
Angle of depression =
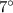
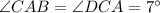 [Alternate angle]
[Alternate angle]
In triangle CAB:
To find AB = x foot.
Using tangent ratio:
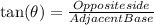
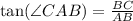
Here, BC = 50 foot and

then;
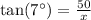
or
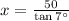

Simplify:
AB = x = 407.217321 foot
Therefore, the boat from the base of the light house is, 407.22'