Question

Solve for x

X+8/5=10-x and


Simplify

3(y+7)^5/(y+7)^4

Answer 1

To solve the equation x + 8/5 = 10 - x, we rearrange to isolate x, yielding x = 21/5 or 4.2. To simplify the expression
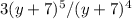`3(y+7)^5/(y+7)^4`, we subtract exponents and obtain the simplified form 3(y+7).

Step-by-step explanation:

Solving the Equation X+8/5=10-x

To solve the equation
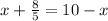`x + (8)/(5) = 10 - x`, we first want to get all the x terms on one side and the constants on the other. This can be achieved by adding x to both sides of the equation and subtracting \frac{8}{5} from both sides.

Doing this gives us:

`2x = 10 - (8)/(5)`

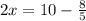
To simplify, we convert 10 to fifths. 10 is the same as
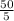`(50)/(5)`, so we have:

`2x = (50)/(5) - (8)/(5)`

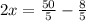
Combining the fractions we get:

`2x = (42)/(5)`

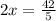
Now, we divide both sides by 2 to solve for x:

`x = (42)/(5) / 2`

`x = (21)/(5)`

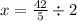
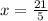
Thus, x equals
`(21)/(5)` or 4.2

Simplifying the Expression
`3(y+7)^5/(y+7)^4`

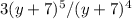
To simplify the expression
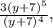`(3(y+7)^5)/((y+7)^4),` we observe that the numerator and the denominator have a common base of (y + 7) raised to a power. We can apply the laws of exponents which state that when dividing like bases, we subtract the exponents. This gives us:

`3(y+7)^(5-4)`

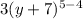
Which simplifies further to:

`3(y+7)`

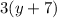
This is the simplified form of the expression.