Question

If someone could help me out with this, I would greatly appreciate it!!

Answer 1

We are given that revenue of Tacos is given by the mathematical expression
`-7x^(2)+32x+240`.

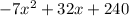(A) The constant term in this revenue function is 240 and it represents the revenue when price per Taco is $4. That is, 240 dollars is the revenue without making any incremental increase in the price.

(B) Let us factor the given revenue expression.

`-7x^(2)+32x+240=-7x^(2)+60x-28x+240\\-7x^(2)+32x+240=x(-7x+60)+4(-7x+60)\\-7x^(2)+32x+240=(-7x+60)(x+4)\\`

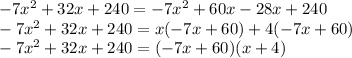
Therefore, correct option for part (B) is the third option.

(C) The factor (-7x+60) represents the number of Tacos sold per day after increasing the price x times. Factor (4+x) represents the new price after making x increments of 1 dollar.

(D) Writing the polynomial in factored form gives us the expression for new price as well as the expression for number of Tacos sold per day after making x increments of 1 dollar to the price.

(E) The table is attached.

Since revenue is maximum when price is 6 dollars. Therefore, optimal price is 6 dollars.