Answer:
(16)
Given the statement: Elmwood st. and oak Dr. are the same distance.
All intersection are perpendicular.
To prove that: Peach Dr. tree is the same distance as Sycamore Ln.
It given that all the intersection are perpendicular which means each interior angles are of
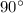
By rectangle properties:
- Each of the interior angles are
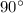
- The opposite sides of the rectangle are equal
- The diagonal of a rectangles bisects each other.
Since, Elmwood st. and oak Dr. are the same distance.
then, by rectangle properties;
Peach Dr. tree is the same distance as Sycamore Ln proved!
6.10
(11)
Given:
 ,
,
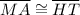
To prove:
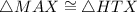
In ΔMAX and ΔHTX
 [Angle] [Given]
[Angle] [Given]
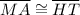 [Side] [Given]
[Side] [Given]
Vertical angle theorem states that angles that are opposite each other.
Since, these angles are formed when two lines cross each other.
And also vertical angles are congruent to each other.
Since,
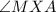 ,
,
 are vertical angles
are vertical angles
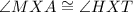 [Vertical angles are congruent]
[Vertical angles are congruent]
AAS (Angle-Angle-Side) theorem states that if two angles and a non-included side in one triangle are congruent to two corresponding angles and a non-included side in another triangle, then those triangles are congruent.
Then, by AAS theorem,
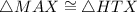
(14)
Given:
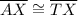 ,
,

Prove that:

In
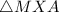 and
and
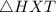
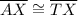 [Side] [Given]
[Side] [Given]
 [Angle] [Given]
[Angle] [Given]
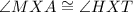 [Vertical angles are congruent]
[Vertical angles are congruent]
ASA (Angle -Side-Angle) theorem states that if two angles and the included side of one triangle are congruent to the corresponding parts of another triangle, then these triangles are congruent.
then by ASA theorem;
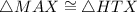
CPCT [Corresponding Part of Congruent triangles are congruent.]
⇒
 [By CPCT] proved!
[By CPCT] proved!