ANSWER
The height of the cylinder is,
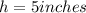
Step-by-step explanation
The volume of a cylinder can be found using the formula,

To make h the subject, we divide through by

This will give us,
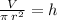
We now substitute
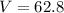
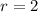
and
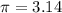
into the given expression for h to obtain,
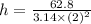
We simplify to get,
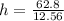

Therefore the height of the cylinder is 5 inches.