Question

There are 32 students in Mrs. Worrell's Classroom. There 12 boys and 20 girls. She needs to select two students to represent her class for a Agora Student Math Department meeting. She will put all students names on a piece of paper and randomly select two names, one after the other. What is the probability that she will select a boy first and then a girl?

Answer 1

15/64 = 23.4 %

Explanation:

Let's consider the first two selections. Let's call B the case in which she extracts a boy and G the case in whish she extracts a girl.

For each selection, the probabilty of event B (selecting a boy) is

`P(B)=(12)/(32)=(3)/(8)`

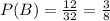
While the probability of event G (selecting a girl) is

`P(G)=(20)/(32)=(5)/(8)`

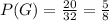
We are asked to find the probability that the first two events are B and then G:

`P(B,G)`

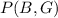
In the first two selections, we have 4 possible combinations:

BB, BG, GB, GG

The probability for each combination is given by:

`P(BB)=P(B)\cdot P(B) = (3)/(8)\cdot (3)/(8)=(9)/(64)=14.1 \%`

`P(BG)=P(B)\cdot P(G) = (3)/(8)\cdot (5)/(8)=(15)/(64)=23.4 \%`

`P(GB)=P(G)\cdot P(B) = (5)/(8)\cdot (3)/(8)=(15)/(64)=23.4 \%`

`P(GG)=P(G)\cdot P(G) = (5)/(8)\cdot (5)/(8)=(25)/(64)=39.1 \%`

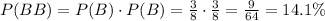
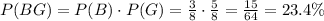
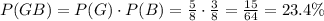
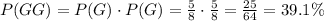
The second one is the probability we are searching for, so the probability that she will select a boy first and then a girl is 15/64, or 23.4%.