Question

Determine which of the following exponential formula(s) represents II and IV in the graph above.

Answer 1

a. α and β

Explanation:

One way to solve this exercise is by analyzing the y - intercept of the functions.

By looking at the graph, we can see that II and IV have the same y - intercept.

All the formulas below depend of the variable ''
`t`'' so if we want to find the y - intercept we only need to replace by ''
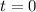`t=0`'' in the formulas.

We can also see that the y - intercept of II and IV will be the lower that the another y - intercept of the functions.

If we replace by
`t=0` :

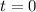(α)(t) =
`10.(1.02)^(t)` ⇒
`10.(1.02)^(0)=10`

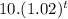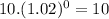
(β)(t) =
`10.(1.05)^(t)` ⇒
`10.(1.05)^(0)=10`

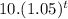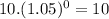
(χ)(t) =
`20.(1.02)^(t)` ⇒
`20.(1.02)^(0)=20`

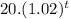(δ)(t) =
`30.(0.85)^(t)` ⇒
`30.(0.85)^(0)=30`

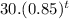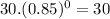
(ε)(t) =
`30.(0.95)^(t)` ⇒
`30.(0.95)^(0)=30`

(Φ)(t) =
`30.(1.05)^(t)` ⇒
`30.(1.05)^(0)=30`

We find that the lowest y - intercept (also equal) are the y - intercept of α and β. Therefore, the correct option is a. α and β

Answer 2

A
`\alpha ,\beta`

Explanation:

Step 1

The first step is to notice that the graphs of II and iv have the same y intercept. This means that we are looking for functions that match the condition that when t=0, the two functions have the same value. The functions
`10(1.02)^t,10(1.05)^t` meet that condition. Additionally the functions
`30(0.95)^t,30(1.05)^t` meet this condition.

Step 2

The other condition that must be met by the 2 functions is that they should both increase with the increase in increasing values of t. the function
`30(0.95)^t` does not meet this condition. This means that only the functions
`\alpha ,\beta` are the only functions that meet this condition.