Question

Which of the following trigonometric equations is false for x?

a) sin x = 2 /√5
b) cos x = -0.1439
c) cos² x + sin² x = 1
d) sec x = √3 / 4
e) tan x = -100

Answer 1

ANSWER


`\sec(x) = ( √(3) )/(4)`


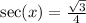
Step-by-step explanation


The correct answer is option D.

The value of the basic cosine function is between negative one and one.

That is for

`f(x)= \cos(x)`
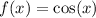
the range of f is

`[-1,1]`

The given equation in option D is


`\sec(x) = ( √(3) )/(4)`

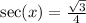
We know that the secant function is the reciprocal of the cosine function.

This implies that,


`\cos(x) = (4)/( √(3) ) = (4 √(3) )/(3)`


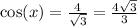
This value is more than 1. It is not within the range of the cosine function, hence it is false because it is not defined.